Revision history [back]

Implementing the AKS primality test

I am implementing the infamous AKS deterministic primality test using SageMath.

My code is as follows:

# AKS Primality Test
def fast_exponentation(a, n):
    """
    Computes a^n fast
    """
    ans = 1
    while n:
        if n & 1 : ans = ans * a
        a = a * a
        n >>= 1
    return ans

# Determines whether n is a power a ^ b, b > 1
def is_perfect_power(n):
    lgn = 1 + ( len( bin ( abs ( n ) ) ) - 2)
    for b in range(2,lgn):
        # we use binary search to check if n root b is an integer
        lowa = 0
        higha = n
        while lowa < higha - 1:
            mida = (lowa + higha) // 2
            ab = fast_exponentation(mida,b)
            if   ab > n: higha = mida
            elif ab < n: lowa  = mida
            else:        return True # mida ^ b
    return False

def AKS_primality_test(n):
    if n == 1: return None
    if n == 2: return True

    # if n is of the form a^b for a > 1 and b > 1 return False
    if is_perfect_power(n): return False
    print("Passed perfect power test")
    # find the smallest integer r such that
    #     a. gcd(n,r) > 1 or
    #     b. [n]_r has multiplicative order > 4 log2(n)^2
    for r in xrange(2,n):
        # if r shares a non trivial factor with n, n is composite
        if gcd(r,n) > 1: return False
        # compute the multiplicative order of n in Z mod r
        ord_r = mod(n,r).multiplicative_order()
        if ord_r > 4*log(n,2)^2: break

    print("min r found! r={}".format(r))

    if r == n: return True

    # We get the ring where we will check for primality
    ZnX = IntegerModRing(n)[x]
    ZnX_div_f = ZnX.quo(x^r-1)

    for j in range(1, 2*log(n,2)*sqrt(r)+2):
        if ZnX_div_f((x+j)^n) != ZnX_div_f(x+j^n): 
            print("The freshman dream test failed for j={}".format(j))
            return False

    print("Passed final test")

    return True

print("271 is prime = " + str(AKS_primality_test(271)))

For the implementation I am following the scheme in page 550 of A Computational Introduction to Number Theory and Algebra, by Victor Shoup (available online in his webpage).

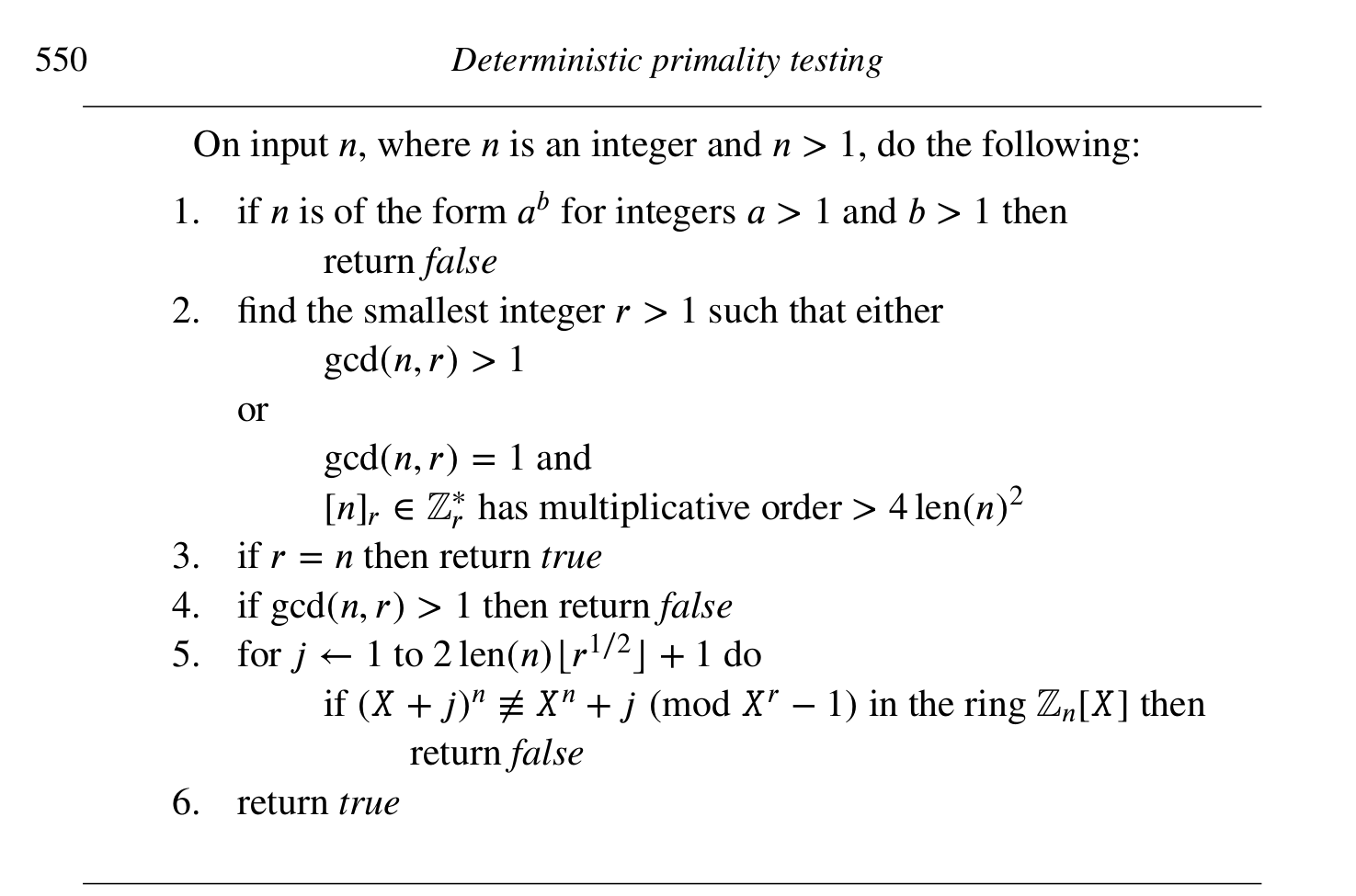
image description

However, the code above, which should test whether 271 is prime, gives us the following output:

Passed perfect power test
min r found! r=269
The freshman dream test failed for j=1
271 is prime = False

This is wrong - 271 is in fact prime. So I assume I am misunderstanding how to use quotient rings in SageMath.

Why does the equality test fail?