Revision history [back]

Say a graph G has nine vertices numbered 1 to 9 and we want the grid layout in the question.

One easily defines a dictionary of positions.

The set_pos method then lets us assign those positions to the graph.

The positions can also be set when creating the graph, using the pos=... optional argument.

For example, define the vertex set.

sage: V = [1 .. 9]

Then the position dictionary:

sage: pos = {v: (n % 3, -(n // 3)) for n, v in enumerate(V)}

Now build a graph with those vertices, no edges, and those positions:

sage: G = Graph([V, []], format='vertices_and_edges', pos=pos)

Plot it:

sage: G.plot()

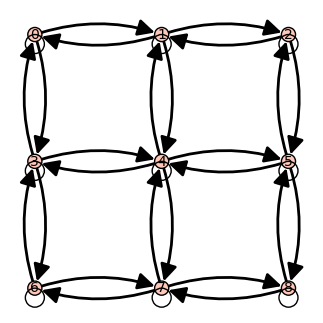
If we start from an adjacency matrix, the vertices will be numbered from 0.

Start from the matrix in the question and remove one of row (chosen so the resulting matrix is symmetric):

sage: A = matrix([[1, 1, 0, 1, 0, 0, 0, 0, 0],
....:         [1, 1, 1, 0, 1, 0, 0, 0, 0],
....:         [0, 1, 1, 0, 0, 1, 0, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 1, 0],
....:         [0, 1, 0, 1, 1, 1, 0, 1, 0],
....:         [0, 0, 1, 0, 1, 1, 0, 0, 1],
....:         [0, 0, 0, 1, 0, 0, 1, 1, 0],
....:         [0, 0, 0, 0, 1, 0, 1, 1, 1],
....:         [0, 0, 0, 0, 0, 1, 0, 1, 1]])

sage: A.nrows(), A.ncols()
(10, 9)
sage: Arows = A.rows()
sage: del Arows[4]
sage: B = matrix(Arows)
sage: B.is_symmetric()
True

Define positions for vertices 0 to 8 and build a digraph from that matrix with those positions:

sage: pos = {n: (n % 3, -(n // 3)) for n in range(9)}
sage: D = DiGraph(B, format='adjacency_matrix', pos=pos)
sage: D.plot()

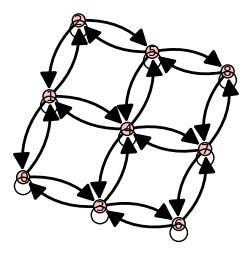
Note that without setting the positions ourselves, the default "spring" layout algorithm would already do a very good job on the grid graph, starting from the corrected matrix.

sage: D = DiGraph(B, format='adjacency_matrix)  # don't set pos
sage: D.plot()

Note that Sage also offers a GridGraph constructor which labels the vertices with their coordinates and positions them in a grid by itself.

sage: G = graphs.GridGraph((3, 3))
sage: G.plot()

Regarding colouring options, the documentations has plenty of examples, and there have also been many questions and answers on Ask Sage which provide nice examples.

Say a graph G has nine vertices numbered 1 to 9 and we want the grid layout in the question.

One easily defines a dictionary of positions.

The set_pos method then lets us assign those positions to the graph.

The positions can also be set when creating the graph, using the pos=... optional argument.

For example, define the vertex set.

sage: V = [1 .. 9]

Then the position dictionary:

sage: pos = {v: (n % 3, -(n // 3)) for n, v in enumerate(V)}

Now build a graph with those vertices, no edges, and those positions:

sage: G = Graph([V, []], format='vertices_and_edges', pos=pos)

Plot it:

sage: G.plot()

If we start from an adjacency matrix, the vertices will be numbered from 0.

Start from the matrix in the question and remove one of row (chosen so the resulting matrix is symmetric):

sage: A = matrix([[1, 1, 0, 1, 0, 0, 0, 0, 0],
....:         [1, 1, 1, 0, 1, 0, 0, 0, 0],
....:         [0, 1, 1, 0, 0, 1, 0, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 1, 0],
....:         [0, 1, 0, 1, 1, 1, 0, 1, 0],
....:         [0, 0, 1, 0, 1, 1, 0, 0, 1],
....:         [0, 0, 0, 1, 0, 0, 1, 1, 0],
....:         [0, 0, 0, 0, 1, 0, 1, 1, 1],
....:         [0, 0, 0, 0, 0, 1, 0, 1, 1]])

sage: A.nrows(), A.ncols()
(10, 9)
sage: Arows = A.rows()
sage: del Arows[4]
sage: B = matrix(Arows)
sage: B.is_symmetric()
True

Define positions for vertices 0 to 8 and build a digraph from that matrix with those positions:

sage: pos = {n: (n % 3, -(n // 3)) for n in range(9)}
sage: D = DiGraph(B, format='adjacency_matrix', pos=pos)
sage: D.plot()

Note that without setting the positions ourselves, the default "spring" layout algorithm would already do a very good job on the grid graph, starting from the corrected matrix.

sage: D = DiGraph(B, format='adjacency_matrix)  # don't set pos
sage: D.plot()

Note that The positions have not been set at the time of creating this graph. Defines pos as above, and running

sage: D.set_pos(pos)
sage: D.plot()

gives the same output as when we defined D = DiGraph(..., pos=pos) above.

Sage also offers has a built-in GridGraph constructor constructor which labels the vertices with vertices by their coordinates coordinates and positions them in a grid all by itself.

sage: G = graphs.GridGraph((3, 3))
sage: G.plot()

Regarding colouring options, the documentations has plenty of examples, and there have also been many questions and answers on Ask Sage which provide nice examples.

Say a graph G has nine vertices numbered 1 to 9 and we want the grid layout in the question.

One easily defines a dictionary of positions.

The set_pos method then lets us assign those positions to the graph.

The positions can also be set when creating the graph, using the pos=... optional argument.

For example, define the vertex set.

sage: V = [1 .. 9]

Then the position dictionary:

sage: pos = {v: (n % 3, -(n // 3)) for n, v in enumerate(V)}

Now build a graph with those vertices, no edges, and those positions:

sage: G = Graph([V, []], format='vertices_and_edges', pos=pos)

Plot it:

sage: G.plot()

If we start from an adjacency matrix, the vertices will be numbered from 0.

Start from the matrix in the question and remove one of row (chosen so the resulting matrix is symmetric):

sage: A = matrix([[1, 1, 0, 1, 0, 0, 0, 0, 0],
....:         [1, 1, 1, 0, 1, 0, 0, 0, 0],
....:         [0, 1, 1, 0, 0, 1, 0, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 0, 0],
....:         [1, 0, 0, 1, 1, 0, 1, 1, 0],
....:         [0, 1, 0, 1, 1, 1, 0, 1, 0],
....:         [0, 0, 1, 0, 1, 1, 0, 0, 1],
....:         [0, 0, 0, 1, 0, 0, 1, 1, 0],
....:         [0, 0, 0, 0, 1, 0, 1, 1, 1],
....:         [0, 0, 0, 0, 0, 1, 0, 1, 1]])

sage: A.nrows(), A.ncols()
(10, 9)
sage: Arows = A.rows()
sage: del Arows[4]
sage: B = matrix(Arows)
sage: B.is_symmetric()
True

Define positions for vertices 0 to 8 and build a digraph from that matrix with those positions:

sage: pos = {n: (n % 3, -(n // 3)) for n in range(9)}
sage: D = DiGraph(B, format='adjacency_matrix', pos=pos)
sage: D.plot()

Note that without setting the positions ourselves, the default "spring" layout algorithm would already do a very good job on the grid graph, starting from the corrected matrix.

sage: D = DiGraph(B, format='adjacency_matrix)  # don't set pos
sage: D.plot()

The positions have not been set at the time of creating this graph. Defines pos as above, and running

sage: D.set_pos(pos)
sage: D.plot()

gives the same output as when we defined D = DiGraph(..., pos=pos) above.

Sage has a built-in GridGraph constructor which labels the vertices by their coordinates and positions them in a grid all by itself.

sage: G = graphs.GridGraph((3, 3))
sage: G.plot()

This gives an undirected graph.

Turning it into a directed graph is easy:

sage: D = DiGraph(G)

This preserves the positions!

One can also relabel the vertices:

sage: D.relabel([1 .. 9])

This also preserves the positions!

To sum up, the easiest way to achieve the graph in the question might be:

sage: D = DiGraph(graphs.GridGraph((3, 3)), loops=True)
sage: D.relabel([1 .. 9])
sage: D.add_edges((i, i) for i in D.vertices())
sage: D

Regarding colouring options, the documentations has plenty the customisation of examples, vertex and there have also been edges labels, start by checking the documentation and/or the many questions questions and answers on Ask Sage which which provide nice instructive examples.